1. Continuity/Discontinuities:
-A continuous function is predictable. It has no breaks in the graph, no holes, and no jumps. it can be drawn with a single unbroken pencil stoke and it makes a good bridge.
-A discontinuous function can have 3 types of discontinuities, which are in two families. The two families are removable and non-removable discontinuities. In the removable discontinuities there is a Point discontinuity(known as A Hole). In the non-removable discontinuity family there are: jump discontinuities, oscillating behavior, and infinite discontinuities. They are in two families because Removable discontinuities have limits and non-removable discontinuities do not have limits.
Removable discontinuities:
|  |
| https://centralmathteacher.wikispaces.com/file/view/math1.JPG/30652173/math1.JPG |
Non-removable discontinuities:
 |
| http://www.math.brown.edu/utra/discontinuities%205.gif |
2. Limits:
-A Limit is the intended height of a function. Sometimes the value of a limit is just approached, meaning the graph never reaches it. A limit exist as long as the height is reached from the left and the right. The right hand limit and the left handed limit must be the same. If a graph does not break at any given x-value then a limit exist. A limit can exist even if the destination is a hole in the graph.
-A limit does not exist at three non-removable discontinuities, which are:
comparing left and rgiht behavior,
unbounded behavior, and
oscillating behavior. When
comparing left and right they both must approach the number. If the left-handed limit and the right-handed limit are not the same then the limit does not exist. One-handed statements must be written explaining how the left and the right are not the same.
Unbounded Behavior exist because of vertical asymptotes. Having vertical asymptotes makes the function approach infinity from the right and negative infinity from the left. When the limit is unbounded because infinity is not a real number. Oscillating behavior is very wiggly at the origin. This type of function does not have a limit because it doesnt approach any single value.
Left and right Limit notation:
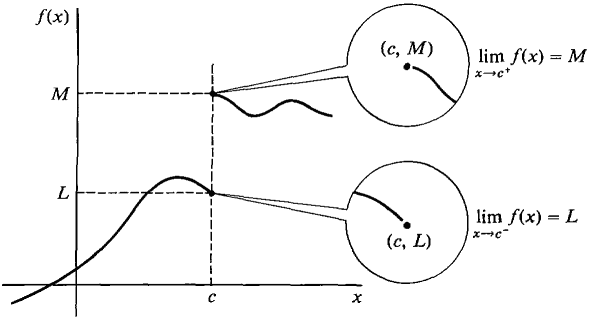 |
| http://www.vias.org/calculus/img/03_continuous_functions-69.gif |
Unbounded Behavior:
 |
| https://apcalckellyanderika.wikispaces.com/file/view/vert_asy.gif/206449186/vert_asy.gif |
|
|
Oscillating Behavior:
 |
| http://webpages.charter.net/mwhitneyshhs/calculus/limits/limit-graph8.jpg |
3.Evaluating Limits:
-Limits can be evaluated numerically, graphically and algebraically. To evaluate them numerically we must use a table. To evaluate them graphically a graph must be used and then we must compare the left and right limits. To do it algebraically there are three different ways:
direct substitution,
factoring, and
rationalizing. With
direct sub. we plug in number. We can get a numerical answer(#), a zero(0/#), the limit does not exist(#/0), and we can get indeterminate form(0/0). If we get indeterminate form that means we must use the other methods to solve the problem. WE ALWAYS USE
DIRECT SUB. FIRST. To use
factoring means that direct sub. did not work. We must factor the top and the bottom to cancel out things that give us zeros, then we can use direct sub.
Rationalizing is used when we multiply the top and the bottom by the conjugate, when using the conjugate we must switch the middle sign.



